When it comes to solving equations and balancing expressions, we can say we are solving algebra. Algebra helps us in finding the value of the unknown in a variety of ways. Algebra is often a difficult to understand for students, and if in creative tasks can be applied to use imagination or, in last resort, appeal to the best paper writing service that will help with papers, mathematics, in turn, requires precise knowledge and an analytical mindset, so now we will try to be as accessible as possible explain linear equations.In this article, let us uncover linear equations in two variables. An equation with two unknown variable quantities and a degree of two is known as a linear equations in two variable. If we talk of a linear equation with one variable, then the linear equation has the greatest power as one of the variables that it contains. Their usual form of writing is y= mx + b.
This sort of problem is resolved using the “slope-intercept form” approach, which involves writing a line in the manner: y = mx + b, in which b represents the y-intercept while m represents the actual slope.
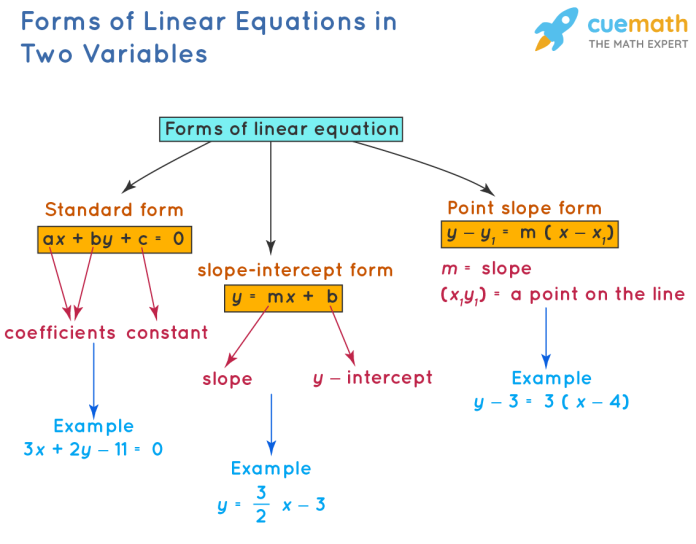
A point where a line passes across the axial direction of a graph is called an intercept. The x-intercept, also known as the lateral intercept, occurs when a point meets the x-axis. And if that point intersects the y axis, it’s known as the y-intercept or vertical intercept. The slope-intercept form of the equation has the benefit of revealing the two most significant characteristics of a line. Like, m is the slope.
An equation is an equivalence that includes variables, according to the definition. The Left Hand Side (LHS) is to the left of the equality sign, while the Right Hand Side (RHS) is to the right of the equality sign. As the equality sign indicates, the values of RHS and LHS are equal in an equation, but only for particular values of the variables involved.
The linear equation in two variables is written as ax + by + c = 0, where the coefficients are a, b, and c, the variables are x and y, and a and b are not equal to zero. The solutions to the equation are x and y in this case. If there is only one solution, the equation is said to be consistent, and if there are no solutions, the equation is said to be inconsistent.
Each solution in the linear equation can be interpreted as the Cartesian coordinates of a point in the Euclidean plane in the case of two variables. A line in the Euclidean plane can be said to be formed by the solutions of a linear equation in two variables, the cluster of all the solutions can be called as each line of a linear equation in two variables. The following are the various methods for solving linear equations in two variables:
- With the help of cross multiplication
- Method of substitution
- By the method of determinants
- Elimination Procedure
- Through the use of graph
In many domains, linear equations in one variable are useful; linear models can also be represented in the slope-intercept form, as in science. This slope-intercept form can be used to find the regression line in statistics.
Math worksheets are a great resource for students to tackle more of these types of problems and improve their skills in solving linear equations. These days, several internet sites provide free worksheets. Cuemath is an example of an online resource where students may access a variety of useful interactive worksheets on this topic. Students may download the worksheets for free from the Cuemath website; they are also printable and provide a fun and engaging approach for students to practice math. These worksheets provide a range of problems that will help students learn how to solve these equations step by step while enhancing upon their analytical and creative thinking skills.